15. Minnows
The one-sided nature of some games in this tournament have led to criticism of the ICC. But data clearly shows that more minnows means more one-sided games
One of the stated reasons for restricting the ongoing World Cup to 10 teams was to ensure that all games were competitive. In previous editions of the World Cup, several games involving non-Test playing nations had been extremely one-sided and not provided too much of a spectacle. Hence, eliminating these “minnows” and having more games between the “big guns” was supposed to make for more interesting cricket overall.
From the looks of it, things haven’t turned out that great. The closest margin in terms of runs has been Pakistan’s 14 run win over England, followed by Australia’s 15 run win over the West Indies. Without doubt, both were excellent games with lots of twists and turns. In terms of chasing, the best we have is New Zealand’s two wicket win over Bangladesh, which was again an excellent game but one where New Zealand let the advantage slip multiple times having been in the driver’s seat.
Apart from this, however, this World Cup has been filled with one-sided games. Afghanistan, possibly reeling from the abrupt change in captaincy, have simply failed to show up and gotten rolled over in all their games. Even Pakistan and Sri Lanka have succumbed meekly in some of their games. South Africa looked listless until they finally encountered Afghanistan. The West Indies, who beat Pakistan so comprehensively in their first game, got beaten by England as comprehensively.
A simulation run by Michale Wagener, who runs “cricket geek” suggests that with high probability we already know the semifinalists.
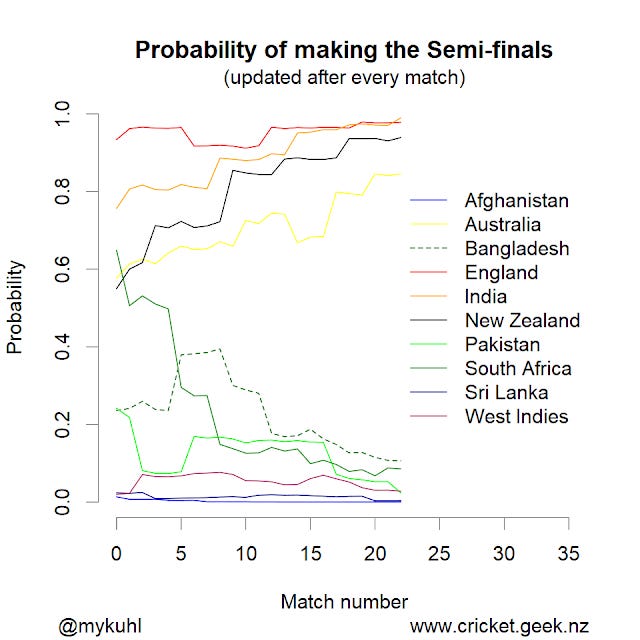
All of this has naturally led to questions regarding the ICC’s wisdom in restricting the tournament to only ten teams. With such a one-sided tournament we might as well have had a more inclusive tournament, goes the argument.
So have matches between the “elite” actually been more interesting than those between the elite and “minnows”? And what about games among minnows (speaking of which, Sri Lanka’s successful chase of Zimbabwe’s 312 in 1992 still ranks among the highest ever successful World Cup chases)?
The last time we discussed interestingness, we used two measures - one was simply the margin of victory and the other based on my model to describe ODI cricket. While the latter might be better since it looks at the entire game rather than just the margin of victory, let’s keep it simple and look at the margin of victory, with one change from last time.
To calculate the margin of victory when the team batting second wins, we will use the old Duckworth Lewis formula (paper version) to extrapolate the scores and calculate the margins (thanks to reader Himanish Ganjoo for supplying the formula). We will calculate the resources remaining at the end of each innings (assuming it was a 50 over innings), and extrapolate the scores in both first and second innings using this. The difference between the two is the margin (this way, even the margin when the team chasing wins is now expressed in terms of runs).
We will consider all teams participating in this year’s World Cup (barring Afghanistan) as the game’s “elite” and call them the “top 9”. The rest of the full ODI members (including Zimbabwe, Afghanistan and Ireland) are “minnows”.
Since the 2015 World Cup, the best games have undoubtedly between a pair of minnows, which have had an average margin of 71 runs. A game between two teams in the top 9 is decided by a margin of 83 runs, while the average margin of victory between a Top9 team and a Minnow is 99 runs.
And this trend has been consistent over time - the games between a top9 team and a minnow have been the most one-sided.
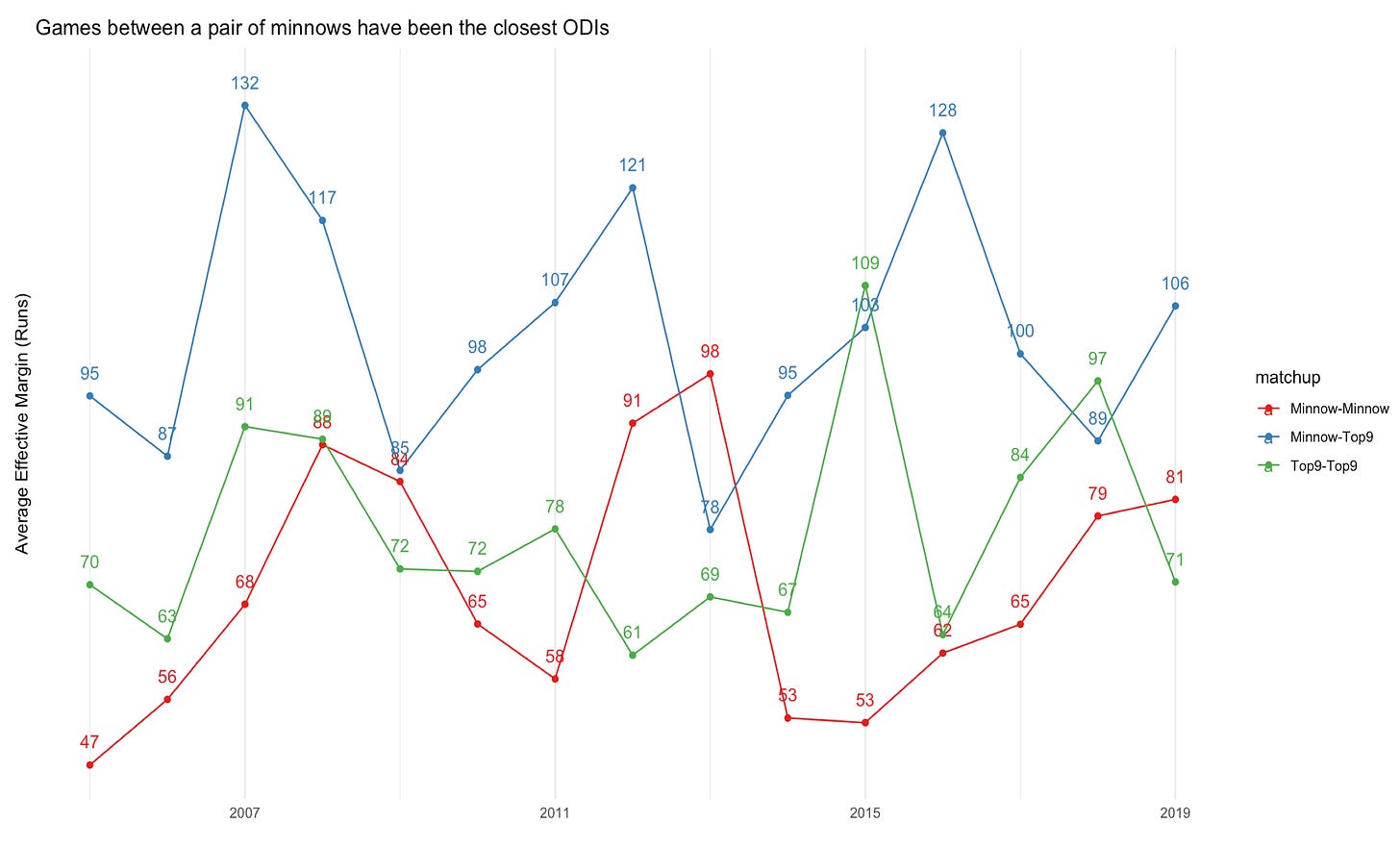
In that sense, the ICC’s contention that excluding minnows from the World Cup in order to produce close matches is rather well-founded (if one were to ignore the possible closeness of games between a pair of minnows). In fact, if we exclude the odd case of a minnow beating a top 9 team (like Afghanistan did multiple times in 2018), we find that the average margin of victory when a top 9 team beats a minnow has consistently been over 100 runs.
The problem is that for the last five editions, World Cup games in general have been uncompetitive. The average (mean) margin of victory so far in this World Cup is 106 runs. This is comparable to the last two World Cups, and less than the 2003 and 2007 World Cups. If we look at the median margin of victory, we find that this World Cup is better than the last four editions, implying that while we’ve had a few highly one-sided games, we’ve also had some reasonably close games.
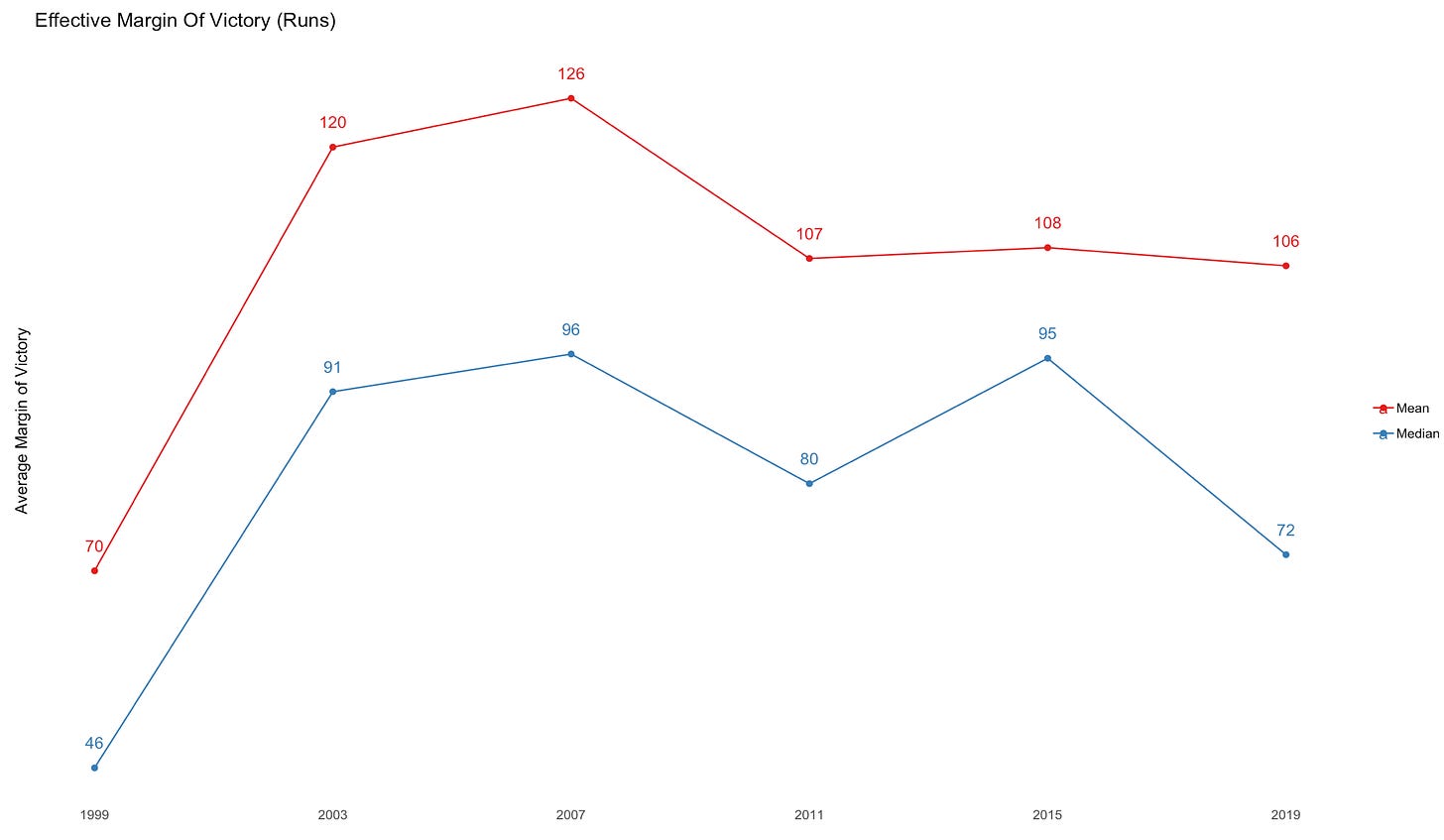
Even if we were to restrict our analysis to the top 9 teams, the numbers don’t look that much different. In other words, World Cup matches in general haven’t been particularly competitive, and this World Cup is no exception.
So while the World Cup hasn’t been particularly exciting (the rain hasn’t helped), it is doubtful if the inclusion of minnows would have resulted in a more exciting or fairer tournament. In terms of fairness, the current format with only two knockout rounds is fairer than the 2011 or 2015 formats which had three knockout rounds (though I would’ve preferred IPL-style playoffs rather than semis and finals).
It remains to be seen if the teams will make the rest of the tournament interesting.

